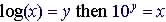
by Examples
Expanded Syllabus
| Chapter 4 : Exponential
and Logarithmic Functions
|
College
Algebra by Examples Expanded Syllabus |
| 4.1 Exponential Functions and Their Graphs
Transformation, k Horizontal: shift left: Vertical: Shift down: Inverse of Exponential
is Logs: Continuous Compounding:
|
A graph of an exponential function is given
by the equation: 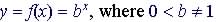
Inc: Increasing exponential function when either x is positive and b is greater than 1 Example: (a)
Dec: Decreasing exponential function when either x is negative or b is less than 1 but greater than 0 Example: (a)
Domain: Some Properties with Radicals - by Examples
(a) (b)
Periodic Compounding: P = initial amount, r = annual rate (decimal) n = number of compound period per year
|
| 4.3 Logarithmic Functions and Their Graphs
Inverse of log is Exponential
|
For 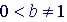 , then
, then 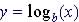 is same as
is same as 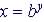
Common Log: Natural Log : Domain: Properties (a) (b) (c) Examples: (a)
|