Examples with Solutions  - ANOVA

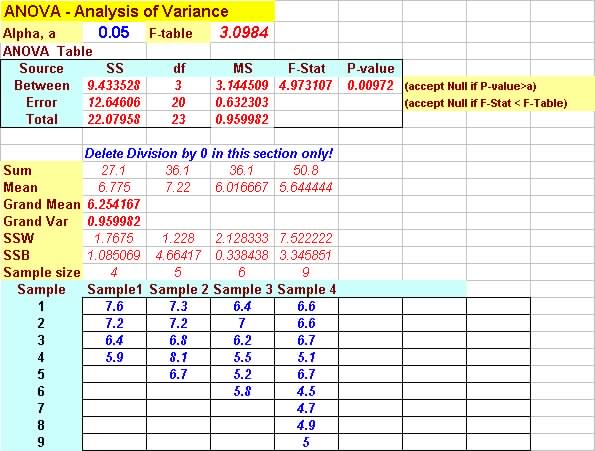
Question 1 The data in the following table represents the starting weekly wages (in hundreds of dollars)
for skilled workers in selected companies in the specified regions.
Do the data indicate a difference in population means? Use a 5% level of significance.
 

Pacific	East North-Central	West North-Central	South Atlantic
7.6	7.3	6.4	6.6
7.2	7.2	7	6.6
6.4	6.8	6.2	6.7
5.9	8.1	5.5	5.1
	6.7	5.2	6.7
		5.8	4.5
			4.7
			4.9
			5

Solution from programs:

Since F-statistics (4.9731) > F-Table (3.0984) Reject the Null Hypothesis that the means are the same.
Also since P-value < 0.01 <0.05 (alpha) RejectH₀

Question 2 (10.31) (a) Complete the following ANOVA table for .

ANOVA Table (only values in Blue were given)
 

Source	Sum of Squares (SS)	Degree of Freedom  (df)	Mean Square	F-Statistics	P-value / F-Table
Between Samples (Explained)	132	4	33	3.3
Within  Samples (Unexplained)	400	40	10
Total	532	44

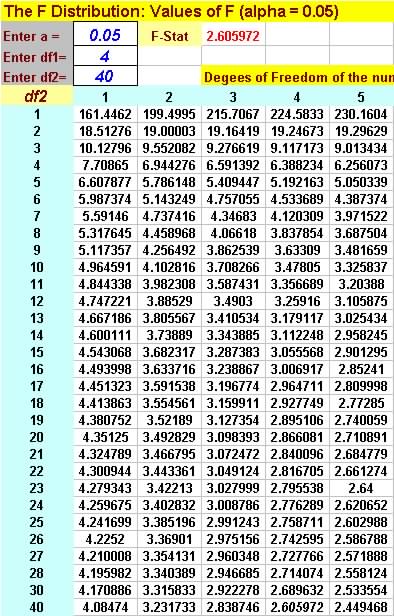
(b) Could you reject the null hypothesis at the 5% significance level?
Since F-statistics (3.3)  > F-table (2.6059) Reject the Null Hypothesis that the means are the same.

Question 3 (10.35) A college administrator claimed that there was no difference in (population) mean college grade point averages
for students coming from three high-school A, B, and C. Use the following data to test the administrator's claim with a 5% significance level.
 

A	B	C
1.9	2.3	2.8
2.3	2.7	2.8
2.8	3.2	2.9
2.4	2.8	3.5
2.5	2.9	3
2.5	2.9

Since F-statistics (5.9942) > F-Table (3.7389) Reject the Null Hypothesis that the means are the same.
Also since P-value < 0.013 <0.05 (alpha) RejectH₀