Answer

Question 1 (5 points) Do Either (a) or (b)

(a) Linear functions problems:

(i) Suppose that f(x) is linear and that f(-2) = 4, f(5) = -3.

Find a formula for f(x). (-2, 4) and (5, -3) -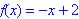

(ii) Suppose that g(x) is linear with slope 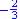passing

through the point (1, 8). Find a formula for g(x).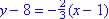

(iii) Suppose that h(x) is linear with an x-intercept of -7 and a y-intercept of -2.

Find a formula for h(x).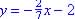

(iv) Suppose that p(x) is linear with x-intercept = 2 and parallel to y = -4x + 2.

Find its formula.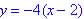

or

(b) Find domain of the function 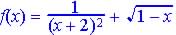The denominator cannot be 0 and the radical cannot be negative. So Domain is all values of x such that Range (not required) is y > 0 (note that there is no value of x that can make y = 0 or negative)

Question 2 (5 points) Piecewise Function

(a) Sketch the piecewise defined function f(x). Use an open circle to represent a point which is not included an solid dot to indicate a point which is on the graph.

(b) What is the value of x when f(x) = 2? Note that f(x) = 2 for both equations 2 and 3. Equation 2: Equation 3: So x = 1.4142 and 2 for f(x) = 2

Figure 4.3