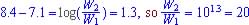Graph A is y = 3x and Graph B is y = 2x since the growth factor b is larger in y = 3x than y = 2x and Graph E is y = e-x since the continuous rate, k is negative.
Graph D is y = log x and Graph C is y = ln x , since ln grows faster than log.
For y = 10x , asym is y = 0 (horizontal)
For y = 2x , asym is y = 0 (horizontal)
For y = log x, asym is x = 0 (vertical)
(a) 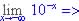 as x gets negative large function becomes 10x
so function becomes large Positively, i.e.
as x gets negative large function becomes 10x
so function becomes large Positively, i.e. 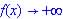
(b) 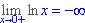
 .
.
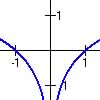
Functions looks like:
|
|
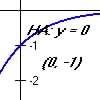
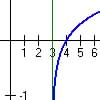
The y-int is (0, -1) Horizontal Asymptote at y = 0 |
Note that for log(x), x > 0
| So x > 0 is
true when x is both positive and negative for x2
Domain: |
|
Note that for log(x), x > 0
| So x > 0 is
true when (x - 3) > 0 or x > 3
Domain: |
|
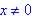
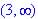
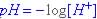 , where H+ is moles per liter
, where H+ is moles per liter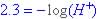
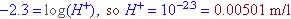
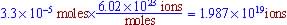
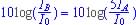
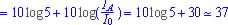

 (log A - Log B = Log (A/B)
(log A - Log B = Log (A/B)